Common Core Geometry – by Kirk

So, the summer has been a great mix of working on Add-Ons for the three courses we currently offer and thinking a lot about Common Core Geometry. I’ve now completed the first four units and a rough course outline that’s been indexed to the CCSS Standards for CC Geometry (as defined by PARCC and refined by NYSED).

I’ve got all of the lessons for those four units posted at this point under our Courses section:

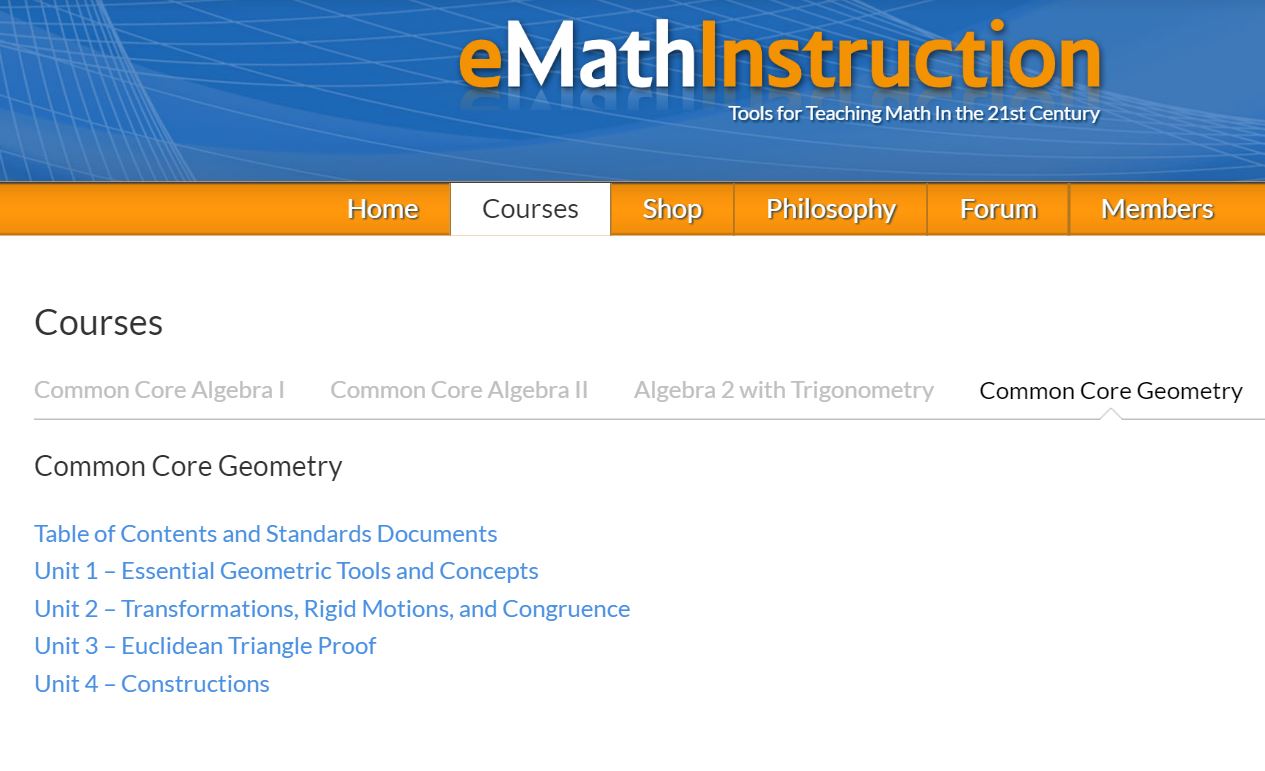
You can find the course outline if you click on the Table of Contents and Standards Documents link. All of the lessons and homework sets posted for the first four units are in rough draft form only. All diagrams that occur in those files were created on our new Efofex software.

The units of the course (at least at this point) are:

Unit 1 – Essential Geometric Tools and Concepts

Unit 2 – Transformations, Rigid Motions, and Congruence

Unit 3 – Euclidean Triangle Proofu

Unit 4 – Constructions

Unit 5 – The Tools of Coordinate Geometry

Unit 6 – Quadrilaterals

Unit 7 – Dilations and Similarity

Unit 8 – Right Triangle Trigonometry

Unit 9 – Circles with and without Coordinates

Unit 10 – The Geometry of Three Dimensions

I don’t think the order of the units is anything too radical, but I wanted to discuss why I have them in this order and my overall thinking at this point. I do want to be clear up front that I believe geometry is the most tactile of all mathematical fields. It is about space and that deserves not just proof and algebraic problems, but also the tools of geometry, which include compass, ruler, straightedge, protractor, and, yes, tracing paper. We currently developing a really good tracing paper that we will begin to sell on our site right after school begins.

Geometry is really all about exploring physical space using tools we develop in mathematics, whether that be transformations, Euclidean logic, or Cartesian coordinates. I began the course in Unit 1 by making sure that students have some of the very basics down, including a really good sense for circles and arcs of circles.

In Unit 2, I wanted to establish certain properties about space by using H. Wu’s rigid motion work with congruence. This is an awesome unit, but probably one of the newer ones in the Common Core. Within this unit, though, we use rigid motions to establish a number of important facts, such as SAS, ASA, and SSS criteria for triangle congruence, facts about the perpendicular bisector of a segment, and parallel line properties.

Unit 3 puts us back on more solid ground with Euclidean triangle geometry proof. I did bring parallel lines back into the mix so that students could also be exposed to AAS and HL methods of proving triangles congruent. We look at many of the classic problems of geometry in this unit, including why the angle bisector represents all points equidistant from the sides of an angle.

In Unit 4 we explore the beautiful world of constructions. I know constructions can be a mystery to students, a set of directions that they need to memorize to carry out the construction. I make sure within every lesson that the constructions have both purpose and are proven by using Euclidean triangle proof.

I haven’t created the remaining units, but want to explain how they will unfold (hopefully). In Unit 5, I am going to introduce coordinate geometry. I wanted to do this relatively early in the course for two primary reasons: (1) so students could have a break from the world of Euclidean reasoning and (2) so that we could use coordinate geometry as well as Euclidean geometry and Wu’s transformation work to explore quadrilaterals (Unit 6) and similarity (Unit 7).

Of course, once we’ve established the concept of similarity in Unit 7, we will naturally move to right triangle trigonometry in Unit 8. Right triangle trig is one of the most applied fields within geometry and we will make sure students understand its basis in similarity and then its application in the real world.

Unit 9, which will come towards the end of the third marking period, will challenge students will the geometry of the circle. But, at this point, we will have a good background in congruence, similarity, the distance formula, and other tools that will allows students to explore the geometry of circles in the Euclidean and Cartesian planes.

Finally, Unit 10 will concentrate on three dimensional geometry as well as measurement and geometric modeling.

I will continue to work on lessons (Unit 5 here I come) for the remainder of the summer and will then begin video work for the first few units once Labor Day is behind us and my own kids have gone back to school (Max and Evie). Hopefully by late September all videos will be done for Units 1 through 4.

Work will continue on the units with pauses in October and November for all sorts of conferences (AMTNYS, AMTNYC, etc). I think, given my current pace, that the first draft of all units should be done by Winter break. I would then expect videos and answer keys to be done with books ready to order by late winter or early spring.

Feel free to give me feedback if you try the materials. If you have questions about the order or any given lesson, just shoot me an email: [email protected]